How much stress do pipes undergo when a liquid flows through them, and how does it depend on the degree of curvature of the pipe?
Bends in pipes are especially crucial, for example in the aortic arch that connects to the left ventricle of the human heart. Piping systems in industrial plants often include bends of 90 degrees or more, can be helical, and can even have 180-degree bends. Fluid mechanists in Sweden have analyzed fluid flow in such pipes with a 180-degree bend. Their research is published in the journal Physical Review Fluids.
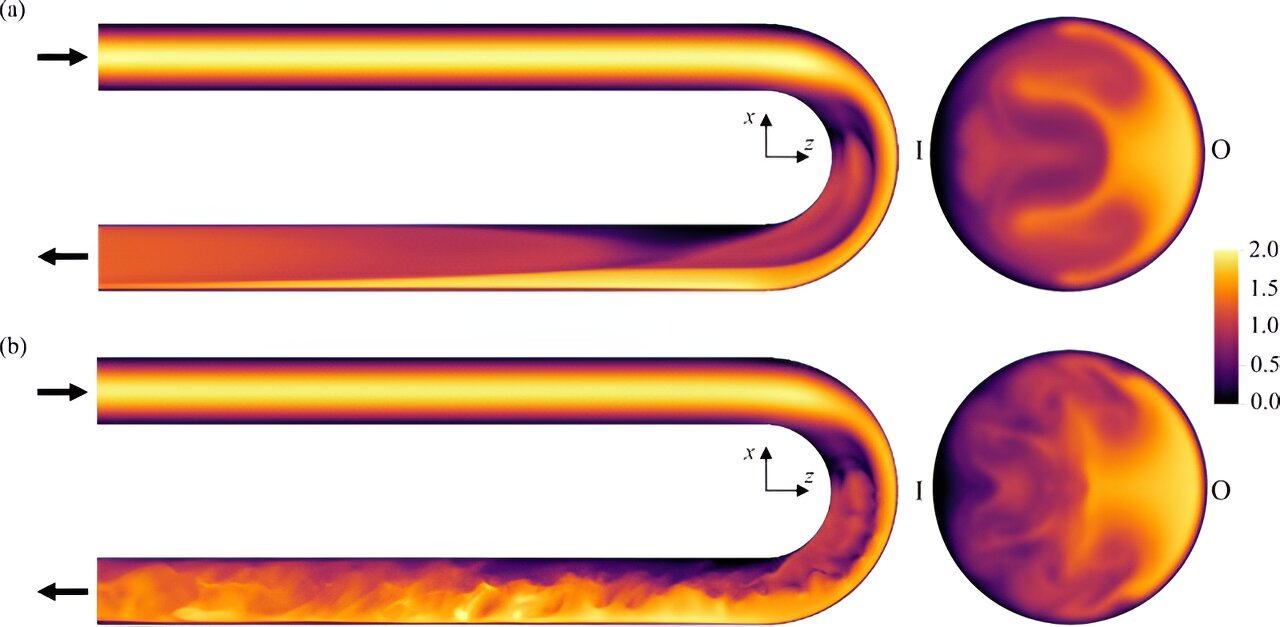
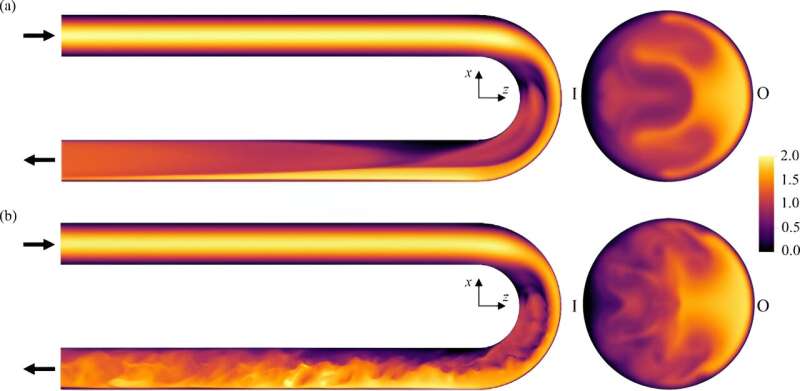
Bends in pipes are different than their straight sections because, in the curved sections, there are outward centrifugal forces due to the inertia of the liquid inside. That force is balanced by a pressure gradient from the outer wall of the pipe to the inner wall. Because the fluid velocities in an imaginary slice through the pipe will not be equal in the curved section—for example, the velocity near the outer wall of the pipe will be greater than near the inner wall—a secondary flow pattern, besides the motion through the pipe, is set up perpendicular to the main flow direction.
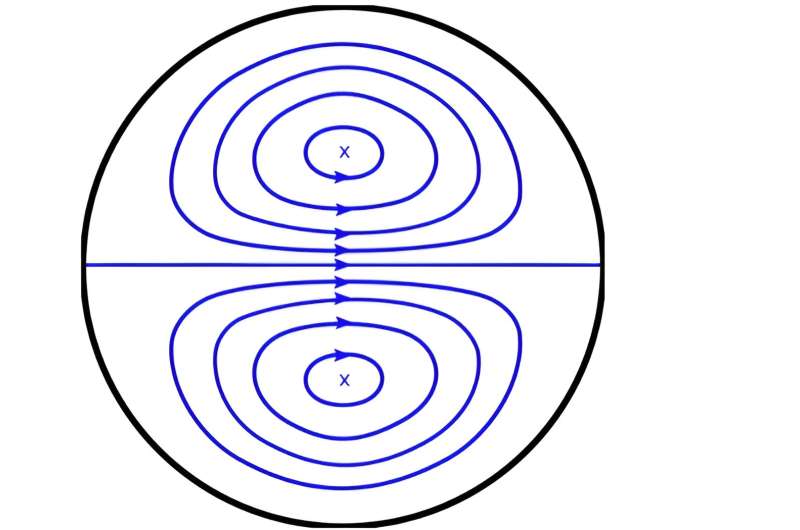
This motion is a pair of counterrotating, symmetric vortices, called Dean vortices, after the British scientist William Reginald Dean, which appear in the first bend in the pipe and can complicate the flow after, for both laminar and turbulent flow.
For a single bend, the internal geometry of the flow can be described by the Dean number, which depends on the radius of the pipe relative to the amount of curvature in the bend, and the fluid’s Reynolds number, which is the ratio of inertial forces to viscous forces within a fluid. Fluids have a critical Reynolds number that characterizes their transition from smooth, laminar flow to turbulent flow, and this can be twice as large as in straight flow. (In fact, turbulent flow from a straight pipe can return to laminar upon entering a spiral section of the pipe.)
Roughly, Reynolds numbers below 2,000 indicate laminar flow, those above 3,500 turbulent flow, with a transition from laminar to turbulent flow occurring somewhere in between. The Dean number measures the intensity of the internal, secondary flow.

Daniele Massaro and colleagues at the KTH Royal Institute of Technology in Stockholm used a refined method to numerically, computationally solve the famously complicated Navier-Stokes fluid equations to analyze the transition (from laminar to turbulent flow) in an idealized pipe with a bend of 180 degrees, comparing their finding to earlier results for elbow (90 degree bend) and toroidal pipes.
Assuming a representative pipe curvature of 1/3—the ratio of the radius of a cross-section of the pipe and the radius of curvature—the group divided the simulated fluid into about 30 million grids, not all uniform. They then solved the equations for the grid points as they changed with time.
By performing a stability analysis—determining the growth of tiny, infinitesimal imperfections that appear in the initial smooth fluid—the calculation determines the changes in the fluid as it rounds the bend. The changes occur through all the vertical cross-sections of the fluid, and along the length of the pipe. In this way, the transition of the flow from laminar to turbulent can be determined.
The intense calculation—for which supercomputers were required, Massaro said, with runs that could take months—found the critical Reynolds number for the transition to be 2,528. This is the region of the Reynolds number of the fluid, regardless of type, where instability arises and the shape of the structure leads to the transition to turbulence. This transition point is also known as a “Hopf bifurcation.” The instability for the 180-degree bend develops much like that of a 90-degree bend. The critical Reynolds number for a 90-degree bend is 2,531, and for a torus, 3,290.
Due to the detailed nature of the instability, pipes with bends greater than 180 degrees are expected to be similar, up to a point. For pipes with shorter bends, the Hopf bifurcation must disappear as the angle of bend approaches zero with the flow remaining laminar. The group estimates the bifurcation vanishes at a bend of about 20 degrees.
Although the research has obvious industrial applications, the extension to the heart isn’t straightforward because of the difference between actual blood and this study’s idealized flow. “Our study helps understand where a sudden transition in the, usually, laminar aortic arch might occur,” said Massaro, the co-author of the study and a graduate student in the department of Engineering Mechanics at the KTH Royal Institute of Technology in Stockholm. “Indeed, the turbulent regime in the aorta can potentially be related to various heart diseases.”
More information:
Daniele Massaro et al, Global stability of 180∘ -bend pipe flow with mesh adaptivity, Physical Review Fluids (2023). DOI: 10.1103/PhysRevFluids.8.113903
© 2023 Science X Network
Citation:
Researchers quantify the onset of turbulence in a pipe bent back on itself (2023, December 8)
retrieved 8 December 2023
from https://phys.org/news/2023-12-quantify-onset-turbulence-pipe-bent.html
This document is subject to copyright. Apart from any fair dealing for the purpose of private study or research, no
part may be reproduced without the written permission. The content is provided for information purposes only.





